Hello all,
Sorry for interrupting, but I have some troubles with plotting bode.
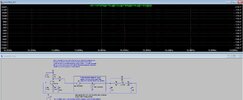
Namely, the picture below shows my oscillator( he is oscillating properly at fruequency of 14.Mhz for which I need to find the transfer function (draw a bode diagram). I need to get the transfer function of the beta branch to see what it looks like and why it oscillates at 14MHz.
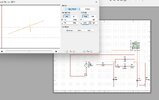
Now, trying, I don't know where I'm going wrong, picture 1 in the attachment is the whole circle, and picture 2 is the B-branch.
View attachment 147040
View attachment 147041
Sorry for interrupting, but I have some troubles with plotting bode.
Namely, the picture below shows my oscillator( he is oscillating properly at fruequency of 14.Mhz for which I need to find the transfer function (draw a bode diagram). I need to get the transfer function of the beta branch to see what it looks like and why it oscillates at 14MHz.
Now, trying, I don't know where I'm going wrong, picture 1 in the attachment is the whole circle, and picture 2 is the B-branch.
View attachment 147040
View attachment 147041
